Q1. What is the mode formula for Class 10?

Ans. The mode represents a specific data value that signifies the value occurring most frequently within a particular sample. It is characterized as the element that appears the greatest number of times among the provided data points. The mode formula is expressed as follows: L + (fm – f1) x h / (fm – f1) + (fm – f2).

Q2 What is the trigonometry formula?

Ans. Trigonometry involves six different functions: tangent, sine, cosine, cotangent, secant, and cosecant. These functions lay the groundwork for a broad spectrum of trigonometry formulas that find application in 10th-grade mathematics.

Maths Formulas for Class 10 Chapter wise

Table of Contents

Maths Formulas for Class 10: Mathematics in Class 10 is a fascinating subject that comprises a lot of formulas for several problem-solving questions. Maths Formulas for Class 10 form a fundamental part of not only class 10 Maths but also form the base of various higher-level maths. Students require a lot of practice to become experts with all the formulas. Each and every question in your class 10 Maths subject, is based on a formula to arrive at a solution.

Class 10 Maths Formulas

Class 10 Maths Formulas include formulas related to real numbers, polynomials, quadratic equations, triangles, circles, statistics, probability and other chapters. Thus it is very crucial to learn and memorize all the Maths Formula for Class 10 to solve the problems very accurately and quickly. Here we have provided a list of all formulas for students that serve as a helpful guide for their board exam preparations and also for other competitive examinations.

Real Numbers Formulas for Class 10

Real numbers include all rational and irrational numbers. They include every number you can use in math, such as whole numbers, fractions, decimals, and numbers that can’t be written as simple fractions (like the square root of 2). They can all be shown on a number line. Real numbers are special because you can add or multiply them, and you’ll always get another real number. This makes them very useful for various calculations and mathematical operations. Essentially, real numbers cover a broad range and are a fundamental part of many math problems.

Integers– These are the numbers that include all the numbers, positive numbers, zero, and negative numbers also i.e. ……-4,-3,-2,-1,0,1,2,3,4,5… so on.
Positive integers– These are: Z+ = 1, 2, 3, 4, 5, ……
Negative integers – These are: Z– = -1, -2, -3, -4, -5, ……

Rational Number – The number which is expressed in the form p/q where p and q are integers and q is a positive integer. For example 3/7 etc.
Irrational Number – The number which can not be expressed in the form p/q. For example π, √5, etc.

HCF = The result of multiplying the least exponent of each shared factor in the numbers.
LCM = The outcome of multiplying the highest exponent of each prime factor present in the number.
HCF (a,b) × LCM (a,b) = a × b

Polynomial Formulas for Class 10

A mathematical polynomial is an expression made up of variables, constants, and coefficients, combined using addition, subtraction, multiplication, and non-negative integer exponents. Polynomials are fundamental in algebra and can have various degrees based on the highest exponent of the variable. They are used to model a wide range of mathematical relationships and real-world phenomena.

The General Polynomial Formula

F(x) = a_nxⁿ + bx^n-1 + a_n-2x^n-2 + …….. + rx +s

If n is a natural number: aⁿ – bⁿ = (a – b)(a^n-1 + a^n-2b +…+ b^n-2a + b^n-1)
If n is even (n = 2a): xⁿ + yⁿ = (x + y)(x^n-1 – x^n-2y +…+ y^n-2x – y^n-1)
If n is odd number: xⁿ + yⁿ = (x + y)(x^n-1 – x^n-2y +…- y^n-2x + y^n-1)

Types of Polynomial

Linea- ax+b=0

Quadratic- ax² + bx + c = 0

Cubic- ax³ + bx² + cx + d = 0

Polynomial Identities

(x + y)²= x² + 2xy + y²
(x – y)²= x² – 2xy + y²
x²– y² = (x + y)(x – y)
(x + a)(x + b) = x²+ (a + b)x + ab
(x + y + z)²= x² + y² + c² + 2xy + 2yz + 2zx
(x + y)³= x³ + y³ + 3xy (x + y)
(x – y)³= x³ – y³ – 3xy (x – y)
x³+ y³ = (x + y)(x² – xy + y²)
x³– y³ = (x – y)(x² + xy + y²)
x³+ y³ + z³– 3xyz = (x + y + z)(x² + y² + z² – xy – yz – zx)
ax² + bx + c = 0 then x = \frac{( -b \pm \sqrt{(b² – 4ac)} )}{2a}

Algebra Formulas for Class 10

(a+b)²= a²+ b²+ 2ab
(a-b)²= a²+ b²– 2ab
(a+b) (a-b) = a²– b²
(x + a)(x + b) = x² + (a + b)x + ab
(x + a)(x – b) = x² + (a – b)x – ab
(x – a)(x + b) = x² + (b – a)x – ab
(x – a)(x – b) = x² – (a + b)x + ab
(a + b)³ = a³ + b³ + 3ab(a + b)
(a – b)³ = a³ – b³ – 3ab(a – b)
(x + y + z)² = x² + y² + z² + 2xy + 2yz + 2xz
(x + y – z)² = x² + y² + z² + 2xy – 2yz – 2xz
(x – y + z)² = x² + y² + z² – 2xy – 2yz + 2xz
(x – y – z)² = x² + y² + z² – 2xy + 2yz – 2xz
x³ + y³ + z³ – 3xyz = (x + y + z)(x² + y² + z² – xy – yz -xz)
x²+ y² =½ [(x + y)² + (x – y)²]
(x + a) (x + b) (x + c) = x³ + (a + b +c)x² + (ab + bc + ca)x + abc
x³ + y³= (x + y) (x² – xy + y²)
x³ – y³ = (x – y) (x² + xy + y²)
x² + y² + z² -xy – yz – zx = ½ [(x-y)² + (y-z)² + (z-x)²]

Quadratic Equation and Formulas for Class 10

ax²+bx+c=0 where a ≠ 0

and

x = -b ± √b² – 4ac⁄2a

Arithmetic Progression Formulas for Class 10

n^th term = a + (n-1) d

S_n = n/2[2a + (n − 1) × d]

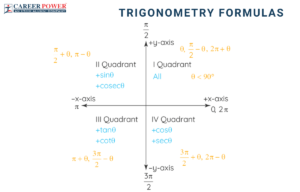
Trigonometry Formulas For Class 10

The basic trigonometry formulas are discussed below for class 10 of all school boards.

sin θ = Opposite Side/Hypotenuse
cos θ = Adjacent Side/Hypotenuse
tan θ = Opposite Side/Adjacent Side
sec θ = Hypotenuse/Adjacent Side
cosec θ = Hypotenuse/Opposite Side
cot θ = Adjacent Side/Opposite Side

Other Trigonometry Formula

sin(90° – θ) = cos θ
cos(90° – θ) = sin θ
tan(90° – θ) = cot θ
cot(90° – θ) = tan θ
sec(90° – θ) = cosecθ
cosec(90° – θ) = secθ
sin²θ + cos² θ = 1
sec²θ = 1 + tan²θ for 0° ≤ θ < 90°
Cosec²θ = 1 + cot² θ for 0° ≤ θ ≤ 90°

Surface Area and Volume Formulas for Class 10

In math, the surface area and volume are important for understanding shapes. The surface area is the total area of all the outer surfaces of a 3D object, like the skin of a ball or box. Volume measures how much space an object takes up, like how much water can fill a bottle. For example, the surface area of a cube is found by adding up the areas of all six faces, and the volume is found by multiplying the length, width, and height. Knowing these helps in real-life tasks like packing and designing objects. Below are some of the standard formulas found in the “Surface Area and Volumes” chapter of the 10th-grade curriculum:

Sphere Formulas

Sphere Formulas
Diameter of sphere	2r
Surface area of a sphere	4 π r²
Volume of Sphere	4/3 π r³

Cylinder Formula

Curved surface area of Cylinder	2 πrh
Area of two circular bases	2 πr²
Total surface area of Cylinder	Curved surface area of Cylinder + Area of Circular bases = 2 πrh + 2 πr²
Volume of Cylinder	π r²h

Cone Formula

Slant height of cone	l = √(r² + h²)
Curved surface area of cone	πrl
Total surface area of cone	πr (l + r)
Volume of cone	⅓ π r²h

Cuboid Formula

Perimeter of cuboid	4(l + b +h)
Length of the longest diagonal of a cuboid	√(l² + b² + h²)
Total surface area of cuboid	2(l×b + b×h + l×h)
Volume of Cuboid	l × b × h

Areas of Circle Formulas for Class 10

Circumference of the circle: 2 π r

Area of the circle: π r²

Area of the sector of angle θ: θ = (θ/360) × π r2

Length of an arc of a sector of angle θ: θ = (θ/360) × 2 π r
(r = radius of the circle)

Probability Formula for Class 10

Probability of an Incident = No. of Favorable Outcomes / Total Number of Possible Outcomes

Statistics Formula for Class 10

In Class 10, the focus of statistics primarily revolves around the analysis of provided data by calculating its mean, mode, and median. Below, you’ll find the formulas for these statistical measures.

Mean

Mean = ∑f x / ∑f

Mode

Mode = L + (f 1– f 0/2f 1– f 0– f 2 ) h

Coordinate Geometry

Coordinate Geometry is a branch of mathematics that introduces you to the concept of using coordinates to describe and work with points, lines, and shapes on a two-dimensional plane, typically represented by a graph with an x-axis and a y-axis. We will examine the coordinate plane and explore the concept of point coordinates, as detailed in the following sections:

Distance Formula

To find the distance between two points, A(x1, y1) and B(x2, y2), on a line, you can use the following formula:
AB = √[(x2 − x1)² + (y2 − y1)²]

Section Formula

For any point p divides a line AB with coordinates A(x1, y1) and B(x2, y2), in ratio m:n, then the coordinates of the point p are given as:
P={[(mx2 + nx1) / (m + n)] , [(my2 + ny1) / (m + n)]}

Midpoint Formula

The coordinates of the mid-point of a line AB with coordinates A(x1, y1) and B(x2, y2), are given as:
P = {(x1 + x2)/ 2, (y1+y2) / 2}

Area of a Triangle

Consider the triangle formed by the points A(x1, y1) and B(x2, y2) and C(x3, y3) then the area of a triangle is given as-
∆ABC = ½ |x1(y2 − y3) + x2(y3 – y1) + x3(y1 – y2)|

Pair of Linear Equations in Two Variables

Linear equation in one variable: ax+b=0 (where a≠0 and a,b are real numbers)
Linear equation in two variable: ax+by+c=0 (where a≠0 & b≠0 and a,b & c are real numbers.)
Linear equation in three variable: ax+by+cz=0 (where a≠0 , b≠0, c≠0 & a,b,c,d real numbers)

Constructions

Construction methods offer insight into the process of creating various types of triangles under specific conditions, employing a ruler and compass with the necessary measurements. Here is the list of important constructions

Determination of a Point Dividing a given Line Segment, Internally in the given Ratio M : N
Construction of a Tangent at a Point on a Circle to the Circle when its Centre is Known
Construction of a Tangent at a Point on a Circle to the Circle when its Centre is not Known
Construction of a Tangents from an External Point to a Circle when its Centre is Known
Construction of a Tangents from an External Point to a Circle when its Centre is not Known
Construction of a Triangle Similar to a given Triangle as per given Scale Factor m/n, m<n.
Construction of a Triangle Similar to a given Triangle as per given Scale Factor m/n, m > n.